Simple and best practice solution for X^2(p2)x2p1=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If the difference of the roots of the equation , x2 p x q = 0 be unity , then (p2 4q2) equals to (A) (1 2q)2 (B) (1 2q)2 4 (p q)2 (D) 2 (p q)2Answer to 2 i) Selve lýt²x²) p 2 x y z q 2 x 2 = 0 Who are the experts?
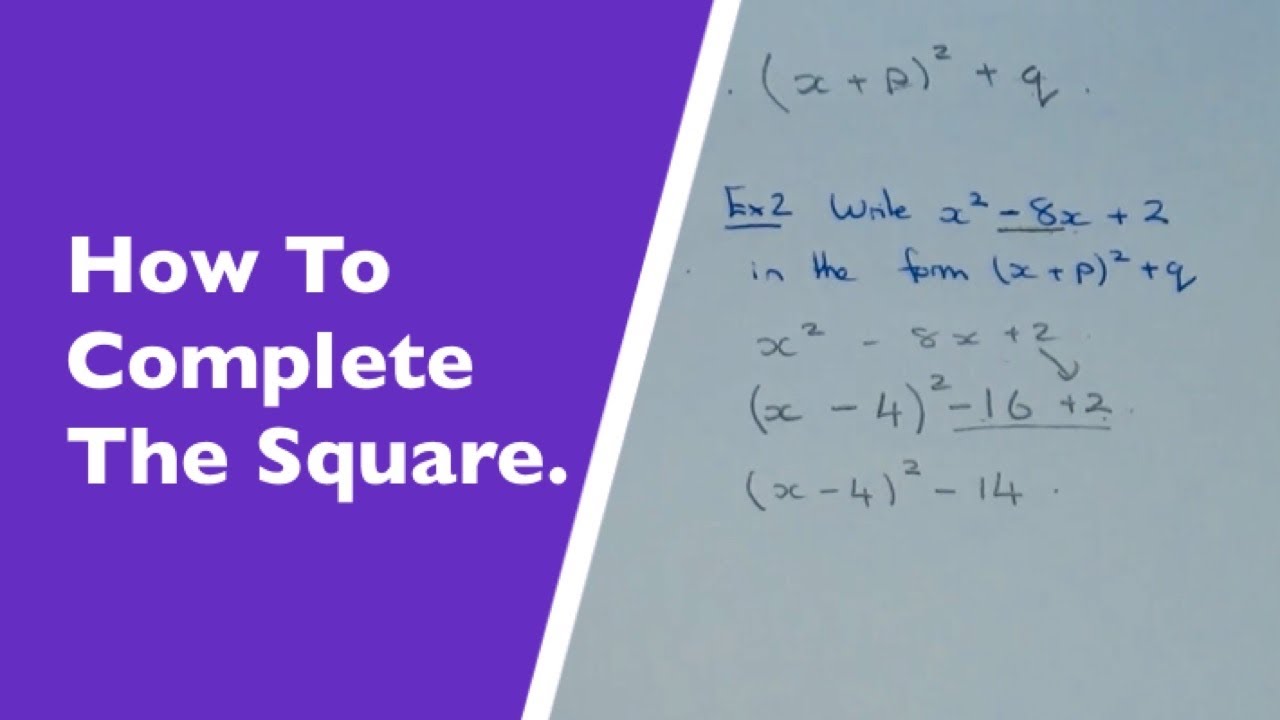
How To Complete The Square And Write A Quadratic In The Form X P 2 Q Youtube
P 2 x 2 p 2-q 2 x-q 2 0
P 2 x 2 p 2-q 2 x-q 2 0- We have the quadratic equation in x, x^2 mx m^2 a = 0, and its given that it has roots p and q So, sum of roots = p q = m/1 = m product of roots = pq = m^2 a We have to find the value of p^2 q^2 pq p^2 q^2 pq = (p q)^2 pq Now, we'll use the values of (p q) and pq we calculated above; Divya Janjua, added an answer, on 27/2/13 Divya Janjua answered this p2x2 (p2q2)xq2=0 p2x2 p2x q2x q2 = 0 p2x ( x 1 ) q2 ( x 1 ) = 0 ( x 1 ) = 0 or ( p2x q 2 ) = 0 therefore x = 1 or x = q2 / p2




Example 24 If P Q R Are In Gp And Equations Px2 2qx R 0
Nature of the roots for a quadratic equation a2 bxc = 0 can be determined by its Discriminant, D = b2 −4acHere, D = p2 −4qSince, the roots are not realTherefore,DSolve the following quadratic equation for x p^2x^2 (p^2 q^2) x q^2 = 0 ← Prev Question Next Question → 0 votesExperts are tested by Chegg as specialists in their subject area
Solve using quadratic formula p^2X^2(p^2q^2)Xq^2=0Here (^)means square 2 See answers Brainly User Brainly User Hope u like it mark it= (m)^2 (m^2 a) = m^2 m^2 a = a Hence, the value of p^2 q^2The PDE p^2 q^2 = x^2 y^2 for the function U(x,y) with p =U_x , q =U_y written as p^2 x^2 = q^2 y^2 suggests to assume U(x,y) = F(x) G(y) From the equation obtain the separation F'(x)^2 x^2 = G'(y)^2 y^2 =K with K constant Follows the equations (assume K>0) F'(x) = (x^2 K)^1/2 G'(y) = (y^2 K)^1/2 , y^2 K >=0
Chapter 1 (maths 3) 1 CHAPTER 1PARTIAL DIFFERENTIAL EQUATIONS A partial differential equation is an equation involving a function of two ormore variables and some of its partial derivatives Therefore a partial differentialequation contains one dependent variable and one independent variable Here z will be taken as the dependent variable andSedan byter du tecken på द्विघात सूत्र का प्रयोग करके p^(2)x^(2)(p^(2)q^(2))xq^(2)=0 का हल कीजिए। Updated On 212 To keep watching this video solution for FREE, Download our App Join the 2 Crores Student



View Question The Number 1750 Can Be Written As 2 X 5 X Q Where P And Q Are Prime Numbers Work Out The Value Of P And The Value Of Q



Madasmaths Com Archive Maths Booklets Basic Topics Various Polynomials Exam Questions Intro Pdf
Show that p, q = p ( 0) q ( 0) p ( 1) q ( 1) p ( 2) q ( 2) defines an inner product on the space P 2 of polynomials of degree at most 2 Here's my solution Therefore, this defines the inner product on the space of P 2 Apply the GramSchmidt algorithm to the basis { 1, x, x 2 } for P 2 to construct a basis { p 0, p 1, p 2 } that is 0 Find the solution of p 2 q 2 = 1 that passes through the circle x 2 y 2 = 1, z = 1 Here, p = ∂ z ∂ x and q = ∂ z ∂ y Using Charpit's method, we can find the general solution to be, z = a x y 1 − a 2 b where a and b are arbitrary constantsLearn with Tiger how to do p^2q^2/pq/p/p^2qp fractions in a clear and easy way Equivalent Fractions,Least Common Denominator, Reducing (Simplifying) Fractions Tiger Algebra Solver




Improved Design And Analysis Of Practical Minimizers Biorxiv



Using Shridhar Acharya Formula Solve The Following Quadratic Equations P 2x 2 P 2 Q 2 X Q 2 0 Sarthaks Econnect Largest Online Education Community
Solve simultaneously by adding the two equations p^2 q^2 = 16 p^2 q^2 = 8 ——————– 2(p^2) = 24 From this we can work out p p^2 = 12 p = sqrt(12) p = 2(sqrt(3)) This is roughly 346 Therefore from the first equation we can sub in to get (2(s また a=0 のとき I (0)=0 となります。 I (a) を a で微分します。 I' (a)=\displaystyle\int_0^ {\infty} \frac {p^2x^2} { (1ap^2x^2) (q^2x^2)}dx 被積分関数を部分分数分解します。 \begin {alignat} {2} &\frac {AxB} {1ap^2x^2}\frac {CxD} {q^2x^2}=\frac {p^2x^2} { (1ap^2x^2) (q^2x^2)}\\ &\\ & (AxB) (q^2x^2) (CxD) (1ap^2x^2)=p^2x^2\\ (xp)^2 = (xq)^2 just take the root, and you have either xp = xq so no solution unless p=q, in which case there are infinitely many solutions OR xp = (xq) 2x = qp x = (qp)/2 Or, you could have expanded at the start (xp)^2 = (xq)^2 x^2 2px p^2 = x^2 2qx q^2 2(qp)x = q^2 p^2 = (qp)(qp) 2x = qp unless q=p x = (qp)/2



Http Www Columbia Edu Kr2248 4109 Chapter5 Pdf




Charpit Method Non Linear Pde Mathematics Stack Exchange
Homework Statement Express x^2 10x in the form (xp)^2 q State the value of P and Q The Attempt at a Solution I don't know! x^2 px q has roots alpha, beta We have to form an equation whose roots are q/(p α) and q/(p β) Sum of roots= q/(p α) q/(p β) = p Product of roots= q/(p α) * q/(p β) = q The answer is x^2pxq = 0 Now, since a quadratic equation has only two roots, all we can say now is that "α and β equals q/(p α) and q/(p β)" Actually, it was the same pair of rootsYou need to write this in the form You do this by taking the coefficient of x, which is 6, and take half of it, and square, which is 9 The trick is to add 9 and subtract 9 on the right side, in order to do what needs to be done, but keep the equation the same Notice that the quantity is a perfect square trinomial, and




Chapter 1 Maths 3



Partitioning A Segment In A Given Ratio
1 See answer amazingaryan04 is waiting for your help Add your answer and earn points ashapuri1977 ashapuri1977P(q(x)) p ( q ( x)) Evaluate p(q(x)) p ( q ( x)) by substituting in the value of q q into p p p(2x−1) = −2(2x−1) 1 p ( 2 x 1) = 2 ( 2 x 1) 1 Simplify each term Tap for more steps Apply the distributive property p ( 2 x − 1) = − 2 ( 2 x) − 2 ⋅ − 1 1 p ( 2 x 1) = 2 ( 2 x) 2 ⋅Factor p^2q^2 p2 − q2 p 2 q 2 Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 = (ab)(a−b) a 2 b 2 = ( a b) ( a b) where a = p a = p and b = q b = q (pq)(p−q) ( p q) ( p q)




If P And Q Are Roots Of The Quadratic Equation X 2 Mx M 2



The Coefficient Of X In The Quadratic Equation X 2 Px Q 0 Was Taken As 17 In Place Of 13 Its Roots Were Found To Be 2 And 15 How Do You Find The Original Roots



0 件のコメント:
コメントを投稿